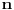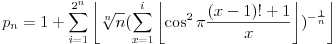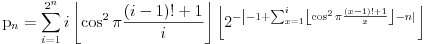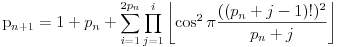da napisem sta znam:
Da li postoji nacin da se odredi naredni prost broj u nizu prirodnih brojeva ?

da ali se prosti brojevi dobijaju nekom jednacinom sa cini mi se 11 clanova i koeficijenata, i sto je najbolje kako menjas n neces dobijati proste brojeve redom.... sve u svemu ovo bi trebala da bude napredna tehnika, ako treba pogledacu sta jos pise o ovome kod cika Merkleta...




I jos nesto : kako matematicki da dokazem da se svaki prost broj moze predstaviti kao 6n-1 ili 6n+1 ?

da bi dokazao ovo mislim da ti treba opsta predstava prostog broja, a to je ovo o cemu sam gore pisao, tj cini mi se da ni ovo nije jednostavno.

P.S:ispravljajte me ako gresim, ovo je sve po secanju...

Bilo koji udžbenik srednjoškolske matematike (ne znam koja godina: 2/3/4) ili matematičke analize (analize? ;-) koji se bavi teorijom brojeva ti može pomoći.

Ako to ne pomogne, onda gledaj ovako: 1 je prirodan broj. 1 se ne može predstaviti kao zbir tri prirodna broja. Znači, 1 se ne može predstaviti kao zbir tri prosta broja. Tvrđenje je netačno.

A zatim, pročitaj pažljivije zadatak, i dodaj i one „nevažne uslove“ koji se u njemu postavljaju. ;-)


Ne bez složenih provera.


Lako — samo primeti da je svaki broj koji nije tog oblika složen, tj. broj koji je nekog od oblika 6n, 6n+2, 6n+3, 6n-2 (ili 6n+4).

Naravno, važno je i znati da se svaki prirodan broj (gde spadaju i prosti) može predstaviti pomoću nekog od oblika 6n+i, gde je i=0,...,5.

Ipak, ovo ne važi za sve proste brojeve, već za one veće od 4.

http://www.utm.edu/research/primes/notes/faq/six.html

kaze :

Perhaps the most rediscovered result about primes numbers is the following:

I found that every prime number over 3 lies next to a number divisible by six. Using Matlab with the help of a friend, we wrote a program to test this theory and found that at least within the first 1,000,000 primes this holds true.
Checking a million primes is certainly energetic, but it is not necessary (and just looking at examples can be misleading in mathematics). Here is how to prove your observation: take any integer n greater than 3, and divide it by 6. That is, write

n = 6q + r
where q is a non-negative integer and the remainder r is one of 0, 1, 2, 3, 4, or 5.

If the remainder is 0, 2 or 4, then the number n is divisible by 2, and can not be prime.
If the remainder is 3, then the number n is divisible by 3, and can not be prime.
So if n is prime, then the remainder r is either

1 (and n = 6q + 1 is one more than a multiple of six), or
5 (and n = 6q + 5 = 6(q+1) - 1 is one less than a multiple of six).
Remember that being one more or less than a multiple of six does not make a number prime. We have only shown that all primes other than 2 and 3 (which divides 6) have this form.



pozdrav.

nemam je, kao sto rekoh to je nesto po secanju, potrazi na internetu, ipak ne mogu da verujem da ti je neko dao problem koji su vekovima matematicari resavali...

Nikako — kada se ovaj problem reši, onda propade sva ovosvetska kriptografija. ;-)

Ono kako se to radi je da kreneš od najvećeg prostog broja koji imaš, i proveravaš svaki sledeći broj da li je deljiv sa ostalim prostim brojevima — prvi koji nađeš koji nije deljiv sa ostalima (svim manjim prostim brojevima), je i sam prost broj.

S tim sto ne moras da proveravas za sve prethodne brojeve vec no najveceg koji je manji ili jednak korenu datog broja.

tacno ali ovaj algoritam je OK za pravljenje programa (cini mi se da takav neki i ima u programskim I na ETF-u) ali mozda to nece njemu koristiti kod matematckog dokaza...

Samo malo da razjasnimo ovo ;)! Da postoji polinom sa 10 clanova (variables), takav da je skup svih prirodnih vrednosti ovoga polinoma jednak skupu svih prostih brojeva. Ovo je jedan od rezultata dobijenih resavanjem desetog hilbertovog problema i Davisove hipoteze iz 50-ih godina. Ukratko posto je skup prostih brojeva efektno izracunjiv (moj slobodan prevod reci computably denumerable, ako sam pogresio molio bih da me ispravite, jer sam los sa nasi terminima), tada postoji i diofantova predstavka (diophantine representation) tog skupa. Posto postoji diofantova predstavka tada po teoremi Julia Robinson iz 60-ih godina postoji i polinom ciji skup pozitvnih vrednosti je jednak skupu koji je efektno izracunjiv. Samo da ne bude zabune ovaj polinom ne moze da kaze ako je dati prost broj n koji je sledeci posle njega.

Svestan sam da ovaj odgovor kasni 2 godine, ali ako je neka uteha formule su večne


Evo nekoliko skoro sasvim beskorisnih Willans-ovih formula koje daju -ti prost broj.
Beskorisnost je posledica upotrebe Wilson-ove teoreme i nekih grubih ocena o broju prostih u datom intervalu.







Znam još jednu formulu u kojoj se ne pojavljuje f-ja , ali je mnogo složena, pa ne smem ni da pokušam da je napišem


Ako uopšte ime svrhe, tražite pa ću da dokažem koju...